Diagnostic GED Practice Test
Use our free GED practice test listed below. You will receive a detailed score report outlining how you did and if you are ready to take the GED.
All GED Practice Tests
If you want to practice for a specific section of the GED, here are the links to our other practice exams:
GED Overview
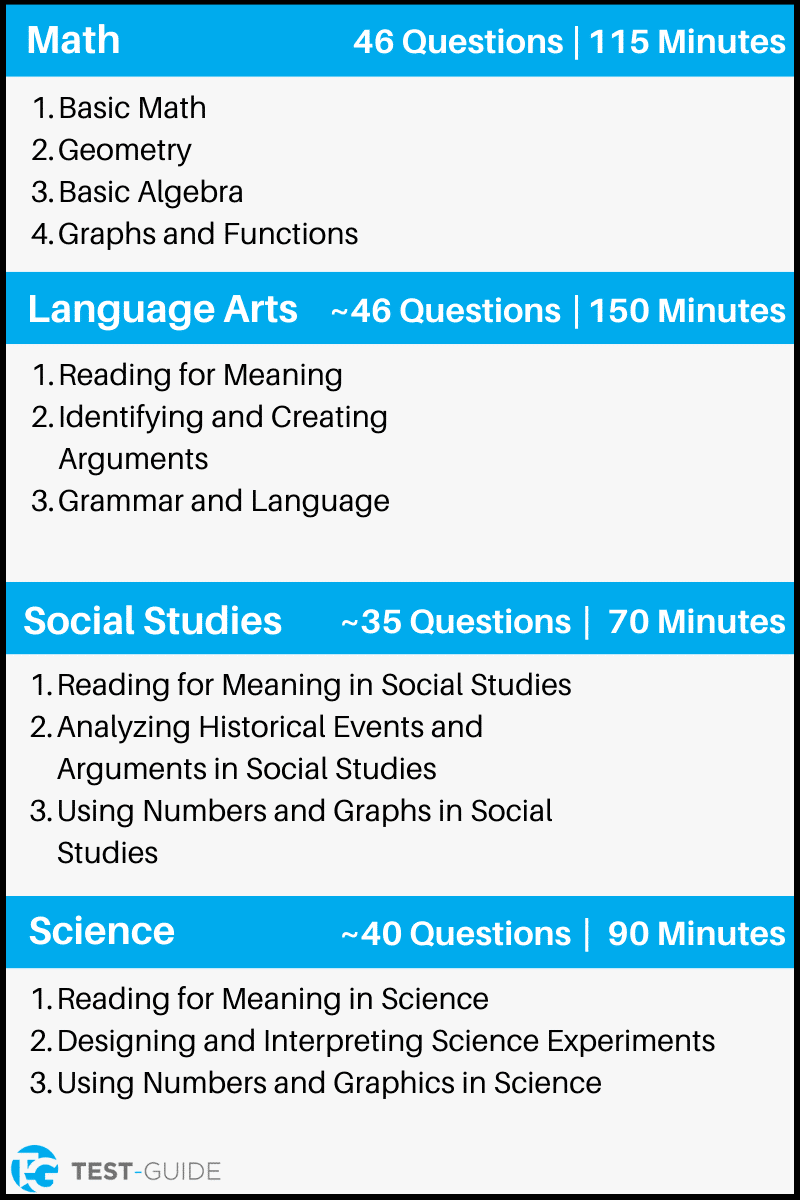
The GED includes 4 different sections:
- Math: 46 Questions – 115 Minutes
- Language Arts: ~46 Questions – 150 Minutes
- Social Studies: ~35 Questions – 70 Minutes
- Science: ~40 Questions – 90 Minutes
Review the image below for a breakdown of the exam. If you want more information on the certificate, read our guide on the GED degree.
GED Printable Practice Tests
We have listed some of our favorite printable GED resources below. You can work your way through each GED PDF and see how you do.
| Resource | Notes |
|---|---|
| Full GED Printable Practice Exam | Take this full GED practice exam and print it out. |
| GED Social Studies Printable Practice Exam | Printable GED exam reviewing social studies. |
| GED Math Printable Practice Exam 1 | Printable GED exam reviewing math. |
| GED Math Printable Practice Exam 2 | Printable GED exam reviewing math. |
| GED Reading Printable Practice Exam | Printable GED exam reviewing reading. |
GED Sample Test – Official Provider of Exam
If you are looking for additional free GED practice tests, you can use the links below. These are other free GED tests online that are beneficial to your studies.
| Resource | Provider |
|---|---|
| GED Math | GEDTS |
| GED Science | GEDTS |
| GED Language Arts | GEDTS |
| GED Social Studies | GEDTS |
GED Practice – Flashcards
If you prefer to practice for the GED with flashcards, we have linked to some of our favorite resources below. We have included a flashcard set for each subject.
| Resource | Provider |
|---|---|
| GED Math Flashcards | Quizlet |
| GED Science Flashcards | Quizlet |
| GED Language Arts Flashcards | Quizlet |
| GED Social Studies Flashcards | Quizlet |
GED Study Guides
If you want to use a GED study guide for your studies, we have linked some fantastic study guides provided by the official provider of the GED.
| Resource | Provider |
|---|---|
| GED Math Study Guide | GEDTS |
| GED Science Study Guide | GEDTS |
| GED Language Arts Study Guide | GEDTS |
| GED Social Studies Study Guide | GEDTS |
How to Best Use a GED Practice Test
Using a free GED practice test is a great way to prepare for your upcoming exam. A GED practice test online will help you narrow down your studies and recognize which areas you may need to spend more time studying.
We recommend the following steps when using our GED sample tests:
- Take the general GED practice exam above to become familiar with the questions and their format
- Take the subject specific free GED tests online that are also linked above to get a better feel for each subject
- Determine which subjects you struggled with the most and spend extra time studying topics, concepts, and questions within those subjects
Some other things you should pay special attention to while using GED practice are the following:
Answer Explanations
You should review all the answer explanations at the end of your practice exam. These answer explanations will help you better understand important concepts and topics. You may even learn a better or faster way of solving a problem.
You should utilize the answer explanations for ALL questions you answered, regardless of if they were answered correctly or incorrectly.
Trends
When taking a practice GED test, do you see any trends or patterns in your results? Are you continuously missing a certain type of math problem or a specific type of language arts problem?
Be honest with yourself and see if you can identify any trends that you can address while studying. This will not only help you study more efficiently, but also help you achieve better GED scores.
Timing
Be aware of how much time you are spending on each question. The GED is a timed exam and many students struggle with the timing aspect of this exam. It is fine to ignore the timing aspect the first couple of times you take a GED sample test, but you should eventually introduce a timing aspect.
Try to find the fastest way possible to solve a problem and make sure it is a repeatable method for you. If you can find methods to solve problems that are both repeatable and speedy, you will be in a great place when taking the actual exam.
Frequently Asked Questions
Is a GED practice test a good way to study for the exam?
A practice GED test is a fantastic way to study for the GED. Not only will you become more familiar with the actual exam, but you will also discover which subjects you need to study the most based on how you do on the practice exam.
Is the GED exam multiple-choice?
The exam is mostly multiple-choice but there are some questions that are not multiple-choice that are included on the exam.
Can you use a calculator on the GED?
You can use a calculator on the exam, but you will not be permitted to use your own calculator. The calculator will be an on-screen calculator.
What score do you need to get your GED?
The GED is scored on a scale of 100-200 points. You will receive a score for each subject. You need at least 145 points on each subject. You can read more about GED scores in our complete guide.

